The Nested Platonic Solids
The nested Platonic Solids can be elegantly represented in
the Rhombic Triacontahedron, as shown in Rhombic
Triacontahedron.
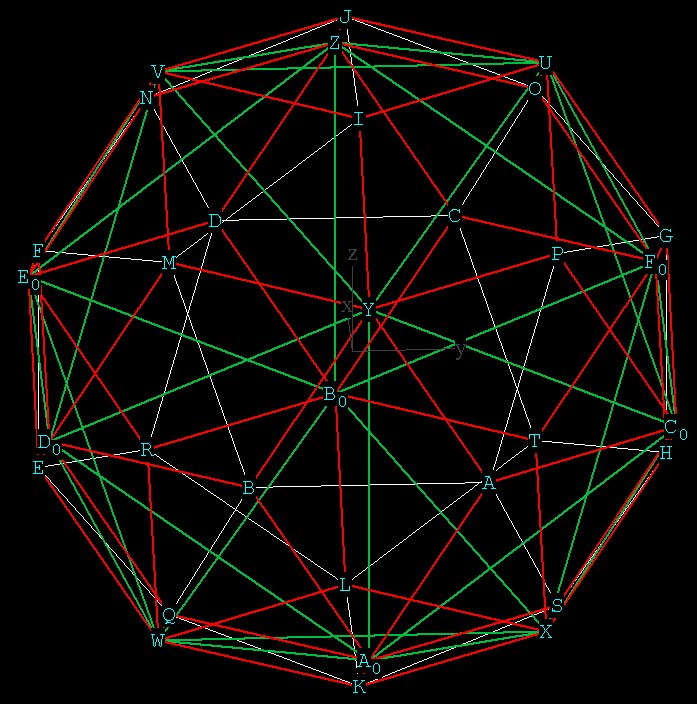
Figure 1 -- the Rhombic Triacontahedron in red with its Phi Ratio rhombi,
the Icosahedron in blue with its equilateral triangle faces, and the Dodecahedron
in black with its pentagonal faces. The Rhombic Triacontahedron is itself a
combination of the Icosahedron and the Dodecahedron, and it demonstrates the
proper relationship between the 5 nested Platonic Solids. You can see how the
edges of the dodecahedron are just the short diagonals of the rhombi of
the rhombic triacontahedron, and the
edges of the icosahedron are the long diagonals of each of the rhombi of the
rhombic triacontahedron. Look at ZDBoC, one of the rhombic faces of the rhombic
triacontahedron. Long diagonal ZB is one of the edges of the triangular face
ZBoFo of the icosahedron, and short diagonal BC is one of the edges of the
dodecahedron face JNDCO.
The vertices of the icosahedron are raised off the faces of the dodecahedron,
so that the icosahedron surrounds the dodecahedron. If you look at point Z, it
rises slightly off the center of the dodecahedral face JNDCO.
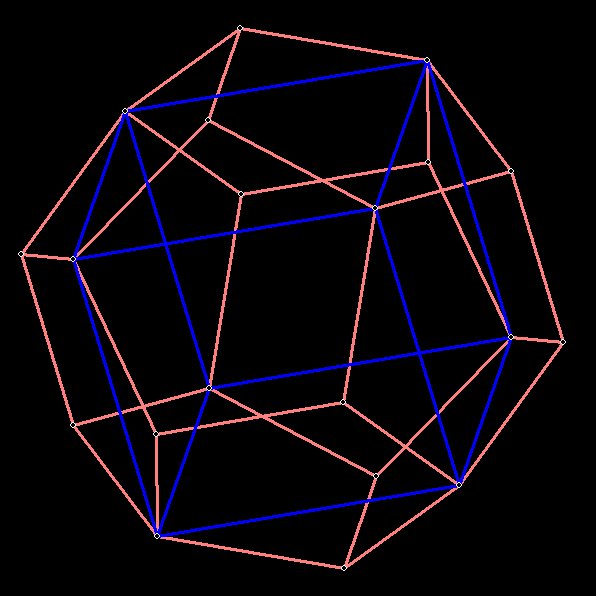
Figure 2 --
The cube fits quite nicely within the dodecahedron, as shown above. The cube
has 8 vertices and 5 different cubes will fit within the dodecahedron. Each
cube has 12 edges, and each edge will be a diagonal of one of the 12 pentagonal
faces of the dodecahedron. Since there are only 5 diagonals to a pentagon,
there can only be 5 different cubes, each of which will be angled 36 degrees
from each other.
(Why is this? Because the diagonals of a pentagon are angled 36 degrees from
each other)
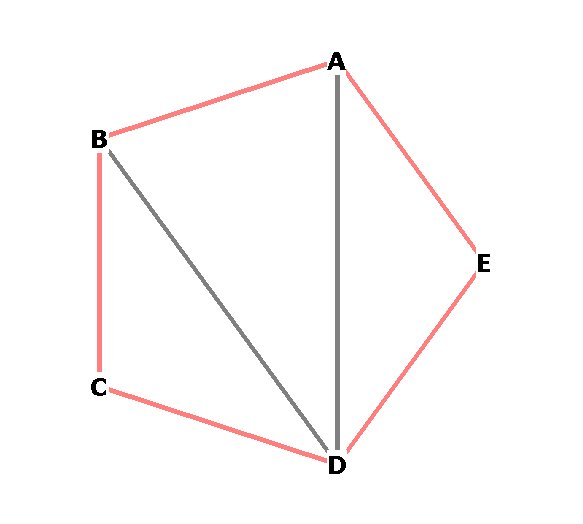
Figure 3 --
The tetrahedron and the octahedron fit nicely within the cube, as shown above.
Figure 3 shows that the octahedron is formed from the intersecting lines of the
2 interlocking tetrahedrons. The edges of the tetrahedrons are just the
diagonals of the cube faces, and the intersection of the two tetrahedron edges
meet precisely at the midpoint of the cube face. If you look at Figure 3 you'll
see how the green and purple lines intersect precisely in the middle of the
cube face, making an "X." At those points you will see one of the
vertices of the octahedron.
The nesting order of the 5 Platonic Solids from largest to smallest, as given
by the Rhombic Triacontahedron, is as follows:
1) Icosahedron
2) Dodecahedron
3) Cube
4) Tetrahedron
5) Octahedron
Here's how the whole thing looks, all enclosed within a
sphere:
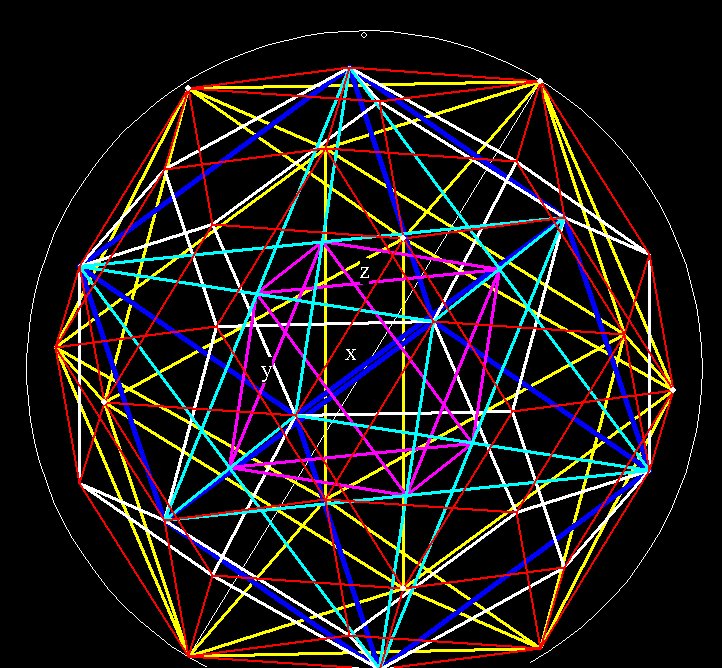
Figure 4 --
The 5 nested Platonic Solids inside a rhombic triacontahedron, surrounded by a
sphere. The Icosahedron in cream, the rhombic triacontahedron in red, the
dodecahedron in white, the cube in blue, 2 interlocking tetrahedra in cyan, and
the octahedron in magenta (for those of you viewing in color). Only the 12
vertices of the icosahedron touch the sphere boundary.
Surprisingly, even though there are 5 Platonic solids, there
are only 3 different spheres which contain them. That is because the 4 vertices
of each tetrahedron are 4 of the 8 cube vertices, and the 8 vertices of the
cube are 8 of the 12 vertices of the dodecahedron.
If we let the radius of the sphere that encloses the octahedron = 1, then what
is the radius of the other two spheres?
Since the octahedron is formed from the midpoints of all of the cube faces, the
sphere which encloses it fits precisely within the cube, like so:
Figure 5 --
The radius of the circle that encloses the octahedron we will arbitrarily set =
1.
The next largest sphere encloses both the cube and the dodecahedron:
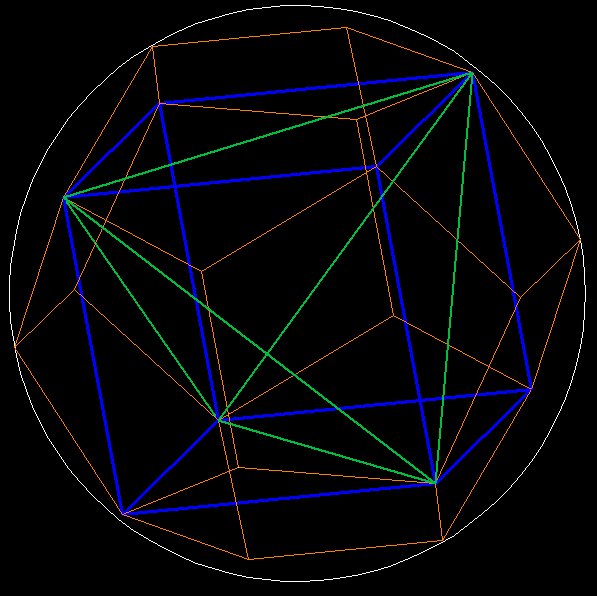
Figure 7 --
The sphere that encloses both dodecahedron and cube.
The radius of this sphere is times the sphere that encloses the
octahedron.
The first two spheres are the in-sphere of the cube and the circumsphere of the
cube.
The outer sphere that encloses the icosahedron (Figure 4) is slightly larger; larger, in fact!
So the radii of the three enclosing spheres is: 1,
Or, 1, 1.732050808, 1.902113033.
Here is a link to an animated GIF that shows all 5 Platonic Solids and the Rhombic Triacontahedron, rotating inside a sphere: http://www.kjmaclean.com/images/Nested.gif
Return to Geometry Home Page The Big Picture Home
